 Department
of
Department
of
Nicholas Kollerstrom's
Newton's 1702 Lunar Theory
This hands-on section shows how to reconstruct past solar and lunar positions, as degrees of celestial longitude, measured from the 'vernal point' as zero. This can involve three different types of time transform, moving between:
* LAT, local apparent time;
* LMT, local mean time;
* GMT or Greenwich mean time ('Universal time'); and
* DT or TDT (Dynamical time), formerly called Ephemeris
time.
The good news is that most of the worked examples we deal with in the early eighteenth-century are given in mean time, often GMT, and the delta-t adjustment to convert to ephemeris time is negligibly small for any times after, say, the mid-seventeenth century.
The last of these is the one which flows evenly (NB, do not attempt to understand this) while GMT or 'universal time' differs in past ages owing to the gradual deceleration of the Earth's rotation, due to tidal friction. The modern 'Dynamical time', formerly Ephemeris Time, is what one feeds into the astronomical computer programs. The first three kinds of time are solar-defined, by the solar days and solar years.
There is a difference, which we may call delta-t,
that bridges the last two of these times. The definitions were set up to
make this of zero or only a few seconds magnitude after 1700, which means
that one can forget it for Newton's lunar theory, but not for those of
Flamsteed or Horrocks. It starts increasing sharply pre-1650: about one
minute in the 1640s, two minutes around Galileo's time in 1600, then over
two hours for Ptolemy's era. (The RGO's Explanatory Supplement to the
Astronomical Almanac gives this function as far back as 1620.) The
conversion to GMT is:
To convert from the local 'apparent time' (sundial time, or 'God's time') -- LAT to LMT -- one requires the Equation of Time, what Flamsteed called the 'Aequation of the Naturall Days'). This function has a maximum value of seventeen minutes and becomes zero four times a year. A program of this is given here (kindly supplied by Bernard Yallop, of the RGO's Nautical Almanac Office: see Hughes, Yallop & Hohenkirk, 1989), that reliably goes back into past centuries.
A lunar and solar-longitude program is also available. One sets it to Julian ('Old Style') or Gregorian ('New Style') time, bearing in mind that Julian time was used by most of Europe in the seventeenth century, though not adopted by Britain until 1752. There is a difference of ten days between these in the seventeenth century and 11 days in the eighteenth. (It may help to remember this by recalling the fact that when the conversion was made in September of 1752 there were riots in the streets of London with the slogan 'give us back our eleven days'.) The equation here is,
So, to reconstruct ancient solar & lunar longitude values for a given time:
Such reconstruction of the historical past was pioneered
by Owen Gingerich and Barbara Welther, and their graphs show error-patterns
of the Nautical Almanac over 1779 - 1787 (1983, p.xi, reproduced
in Wilson, GHA, 1989 p.187-9). But, these show errors of between one and
two minutes of arc for lunar longitude, as would have been of little use
for finding the longitude, whereas I found that the Nautical Almanac
of 1765 had an error of less than one-third this amount. The low accuracy
of this reconstruction, by Gingerich and Welther, could just have been
due to lack of a decent Equation of Time.
Below are three additional worked examples for the lunar
theories:
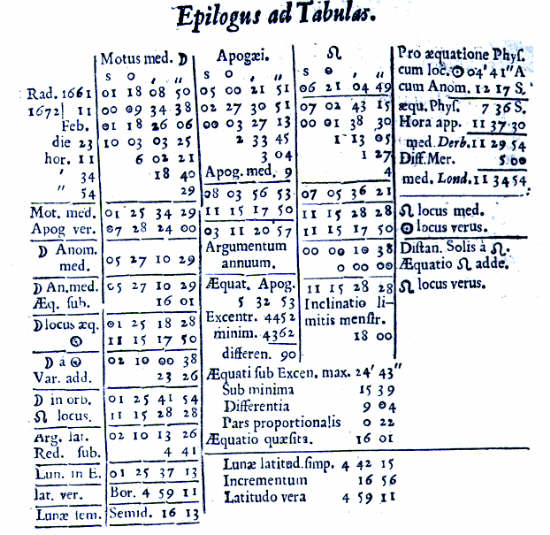
This calculation begins with mean motion (Mot. med.), subtracts an annual equation (Argumentum annuum), then the equation of centre (use Flamsteed program to locate this), then the variation (Var. add), and then the reduction (Red. sub.). The answer is given as Lun. in E. (i.e., the Moon's longitude in the ecliptic).
For more on Horrocks, click here.

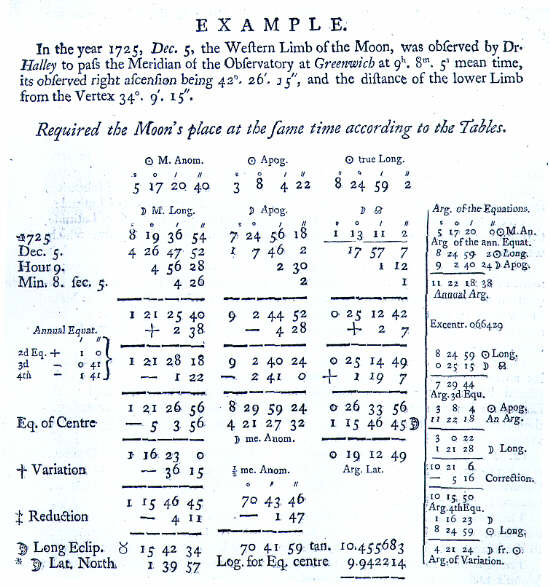
The seven steps of Newton's lunar theory are clearly discernable.
For more on LeMonnier, click here.

Halley slightly adjusted Newton's mean motions. He also slightly simplifed the second equation.
Also note this is close to a lunar eclipse, as the Sun and Moon are in opposition.
For more on Halley, click here.